本文共 528 字，大约阅读时间需要 1 分钟。

【MySQL学习篇】—对 库 的 增删查改

创建 名字为 test 的数据库

注释以 ‘ # ’ 标记

# create database 库名; CREATE DATABASE test;#创建一个库，并且 指定编码格式#create database 库名 character set utf8/gbk;CREATE DATABASE test CHARACTER SET utf8;

删除 名字为test的数据库

# drop database 库名;DROP DATABASE test;

查询

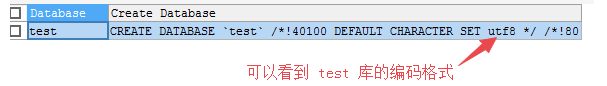
查询指定库的详细信息

#show create database 库名;SHOW CREATE DATABASE test;

查询MySQL下有多少个数据库

SHOW DATABASES;

查询当前用户连接的是哪个数据库

SELECT DATABASE();

查看指定数据库有哪些表

SHOW TABLES;

选中指定数据库进行操作

# use 库名use test;

修改

修改指定库编码

#alter database 库名 character set 新编码;ALTER DATABASE test CHARACTER SET gbk;

转载地址：http://ghsl.baihongyu.com/